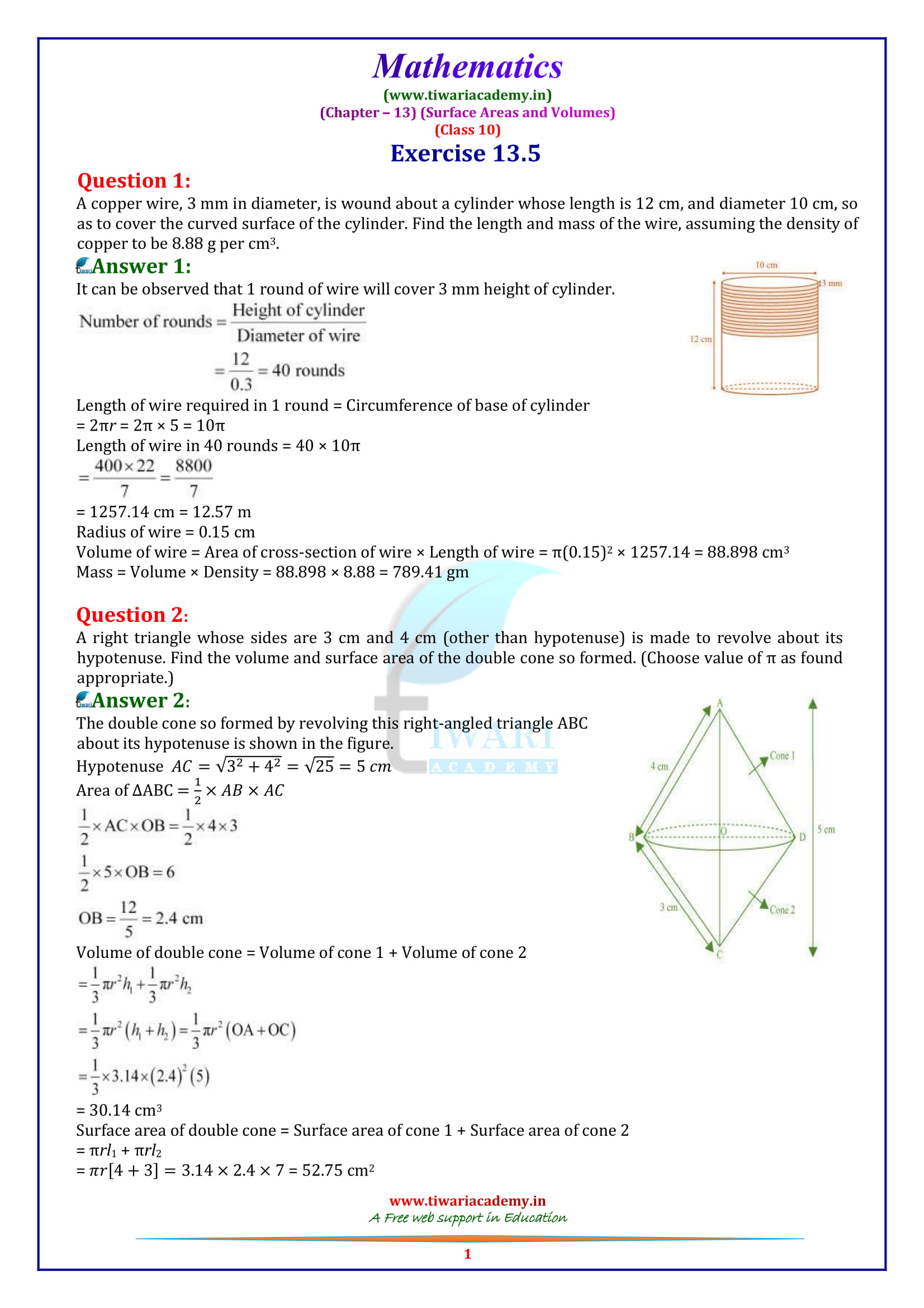
NCERT Solutions for Class 10 Maths Chapter 13 Exercise 13.5 in English Medium and Hindi Medium for all the student of UP Board, MP Board, Uttarakhand and Bihar board, who are following NCERT Books. Surface Areas and Volumes Exercise 13.5 is an Optional Exercise Click Here to see the Exercise 13.1 or Exercise 13.2 or Exercise 13.3 or Exercise 13.4 online.
NCERT Solutions for Class 10 Maths Chapter 13 Exercise 13.5
If you need solutions in Hindi, Click for Hindi Medium solutions of 10 Maths Exercise 13.5

Class 10 Maths Exercise 13.5 Solutions in Hindi Medium

To get the solutions in English, Click for English Medium solutions.
About 10 Maths Chapter 13 Exercise 13.5
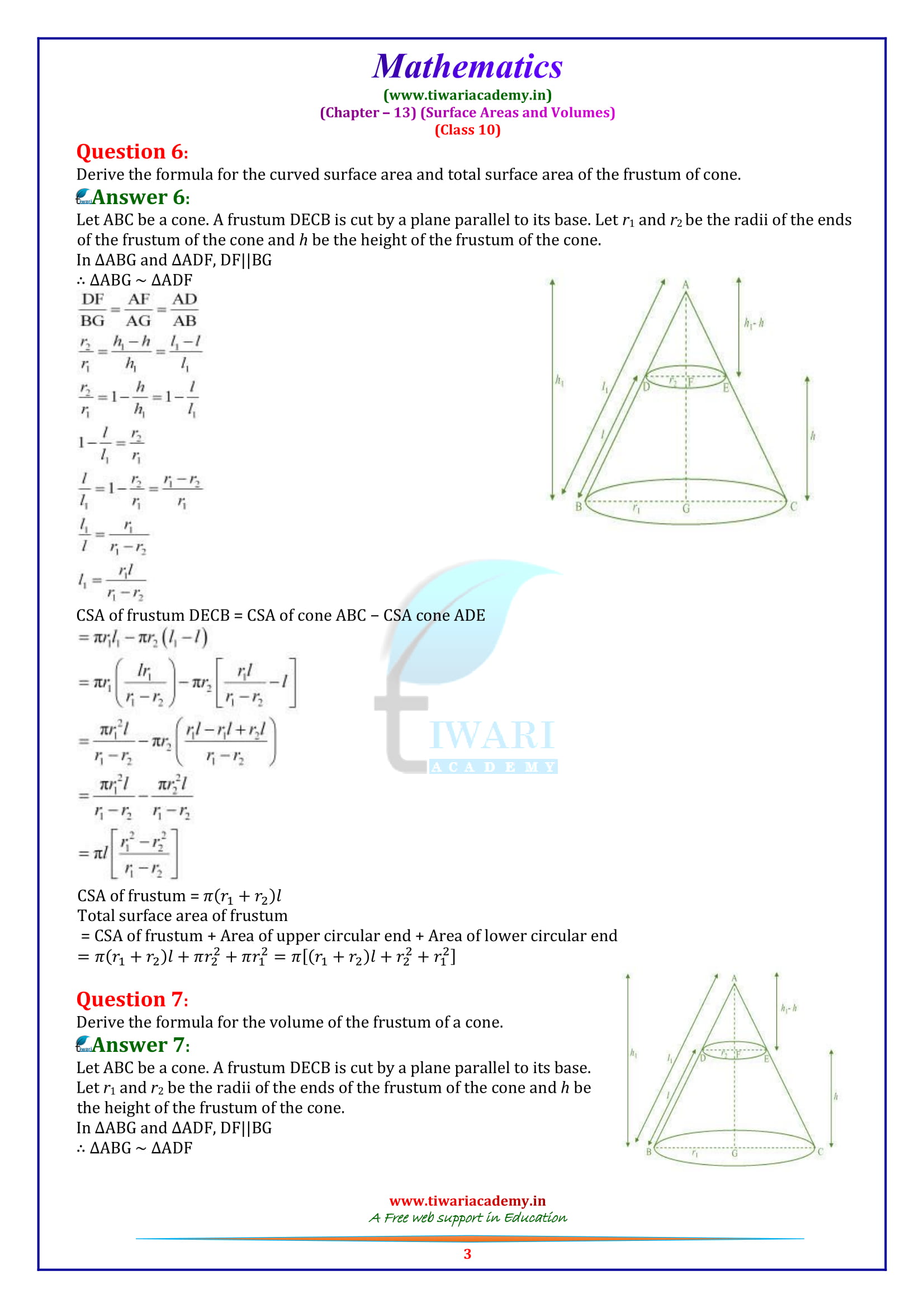
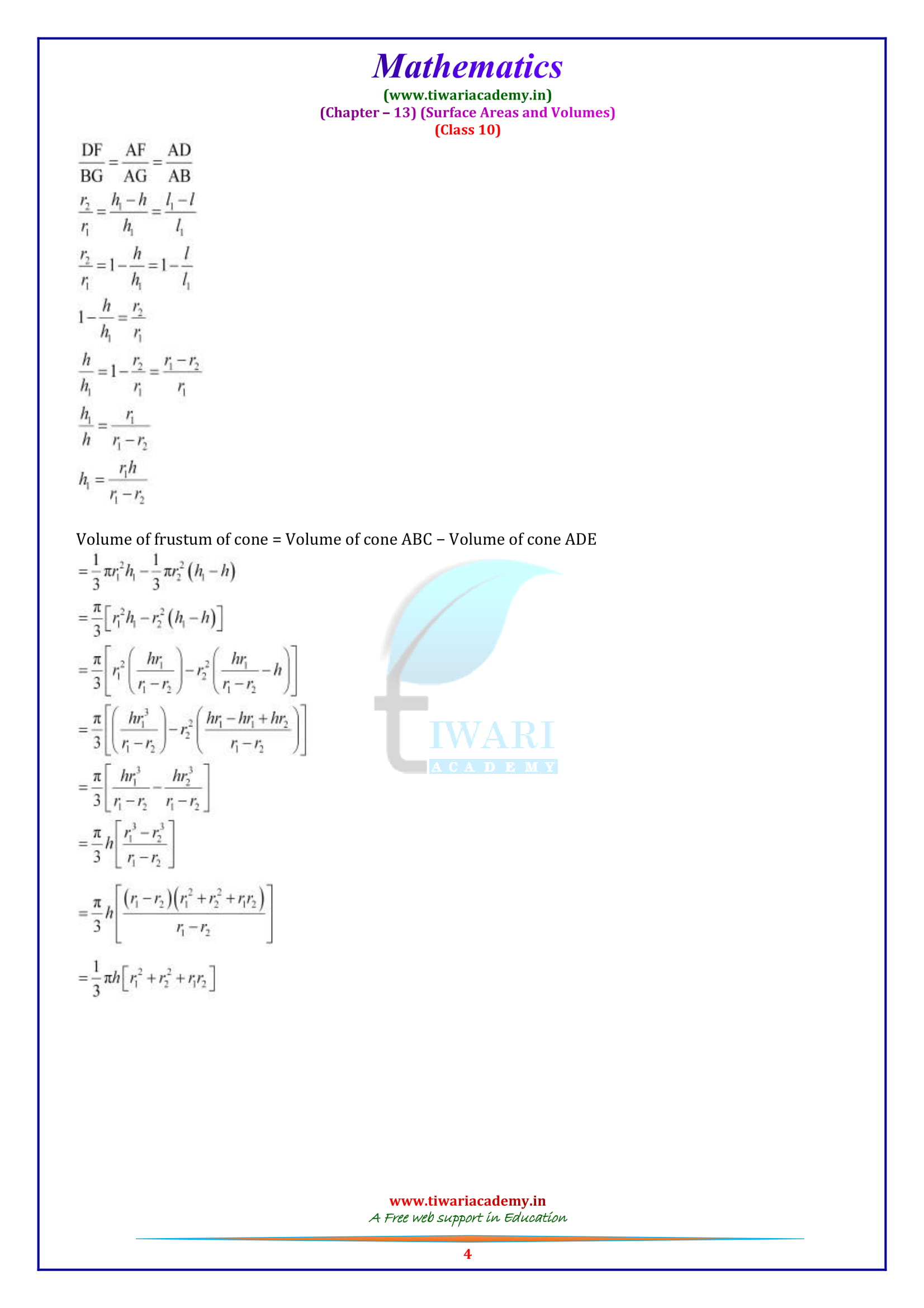
In 10 Maths Exercise 13.5 Most of the questions are based on concepts. Question number 6 and 7 are basically the derivation of the formula of frustum. In NCERT Exemplar of class 10 Maths, some questions are given on the basis of question 6 and 7. The question number 2 can be down in two different methods, only one method is mentions in the solutions given above.
Important Questions for Practice
- Twelve solid spheres of the same sizes are made by melting a solid metallic cylinder of base diameter 2 cm and height 16cm. Find the radius of each sphere.
- A bucket is in the form of a frustum of a cone and holda 28.490 litres of water. The radii of the top and bottom are 28 cm and 21 cm respectively. Find the height of the bucket.
- A cone of radius 8cm and height 12cm is divided into two parts by a plane through the mid-point of its axis parallel to its base. Find the ratio of the volumes of the two parts.
- Water is flowing at the rate of 2.52 km/hr. through a cylindrical pipe into a cylindrical tank, the radius of whose base is 40 cm. If the increase in the level of water in the tank, in half an hour is 3.15m, find internal diameter of the pipe.
- A container, shaped like a right circular cylinder, having diameter 12cm and height 15 cm is full of ice-cream. this ice-cream is to be filled into cones of height 12 cm and diameter 6 cm, having a hemispherical shape on the top. Find the number of such cones which can be filled with ice-cream.