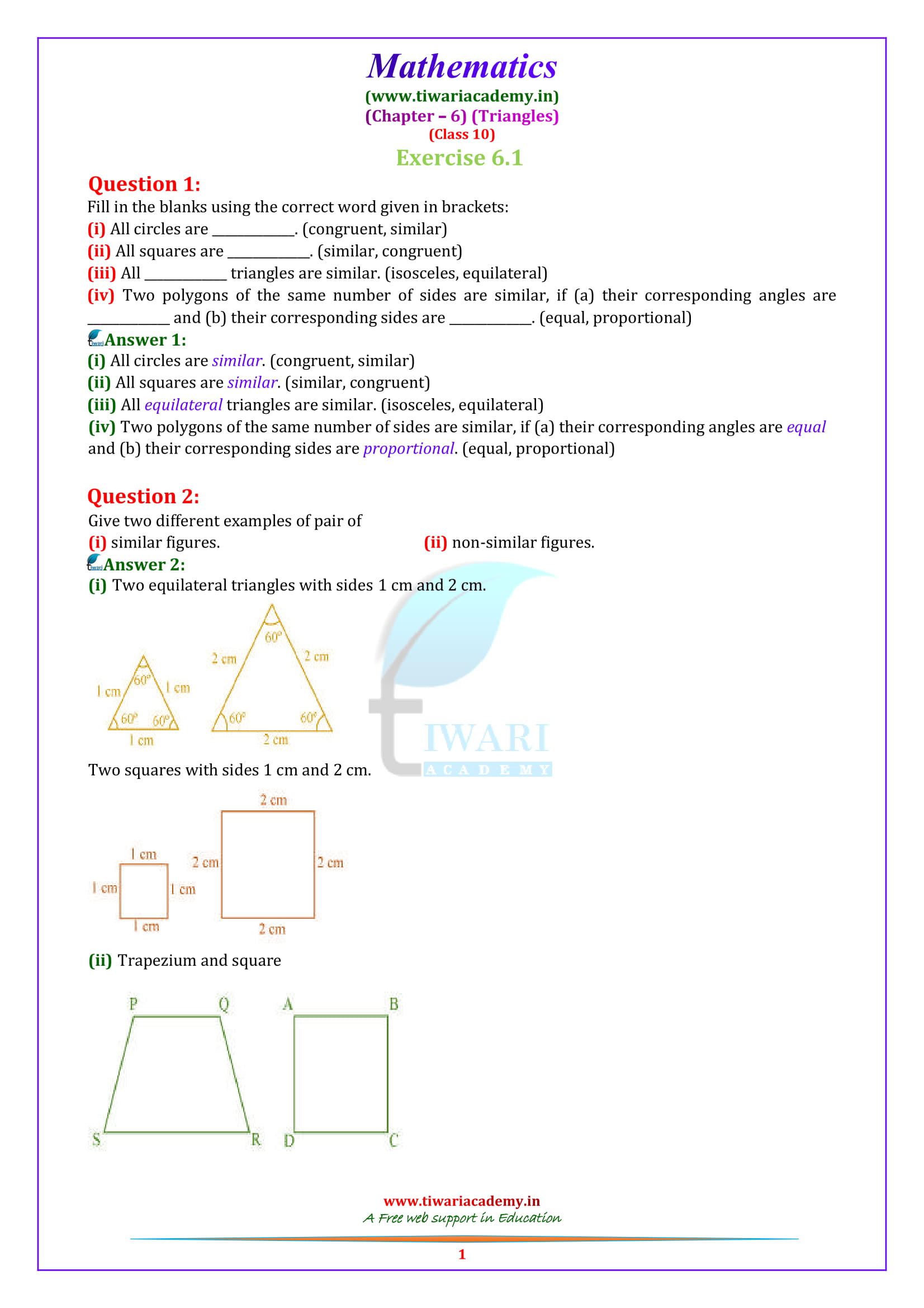
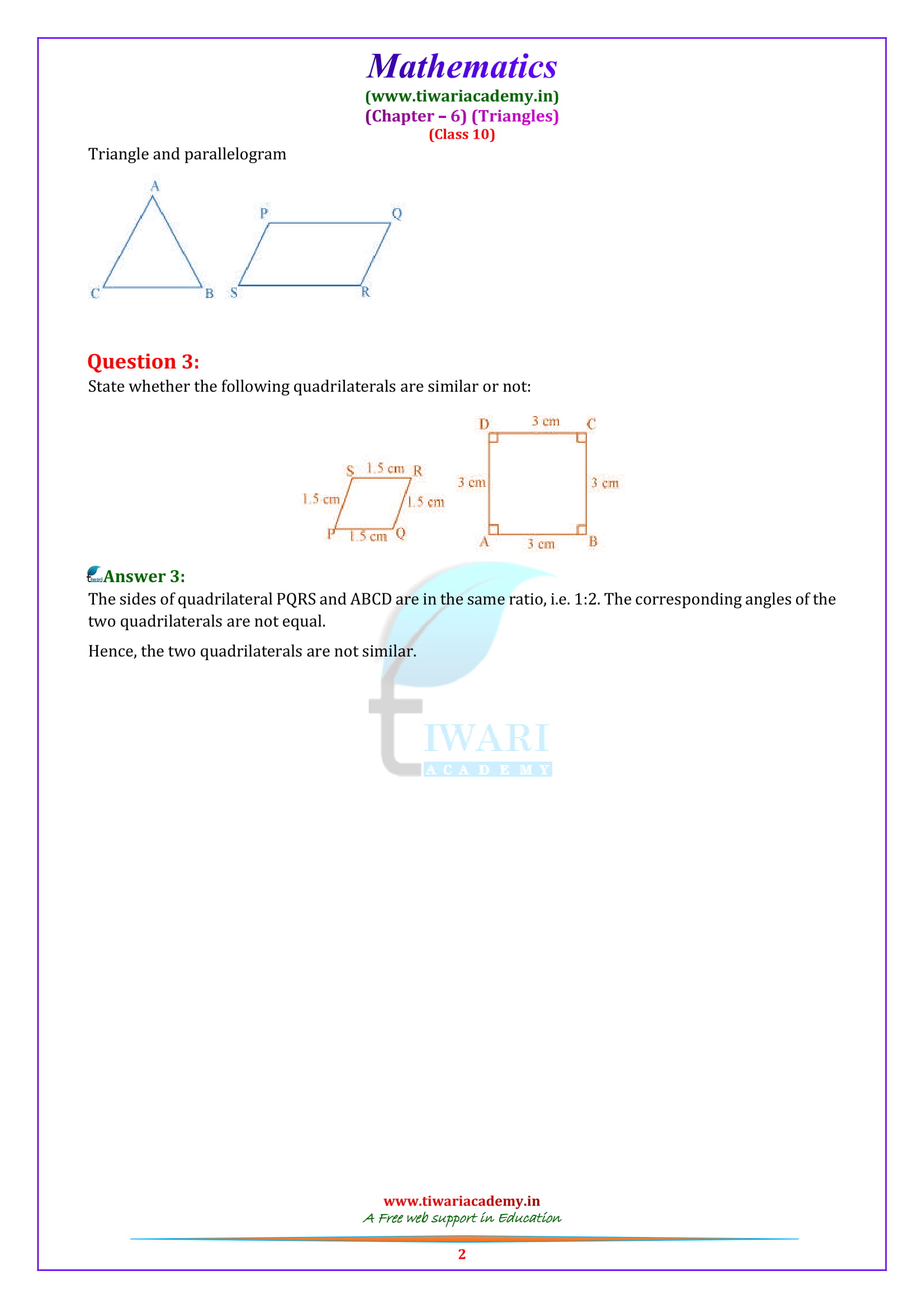
Free download NCERT Solutions for Class 10 Maths Chapter 6 Exercise 6.1 Triangles in English Medium and Hindi Medium form. NCERT Solutions for class 10 based on latest CBSE Curriculum for 2020 – 2021. Download Exercise 6.2 or Exercise 6.3 or Exercise 6.4 or Exercise 6.5 or Exercise 6.6 in PDF form or study online without downloading.
NCERT Solutions for Class 10 Maths Chapter 6 Exercise 6.1
If you need solutions in Hindi, Click for Hindi Medium solutions of 10 Maths Exercise 6.1
Class 10 Maths Exercise 6.1 Solutions in Hindi Medium


To get the solutions in English, Click for English Medium solutions.
The Proof of the following theorems can be asked in the examination:
(i) Basic Proportionality Theorem: If a line is drawn parallel to one side of a triangle to intersect the other sides in distinct points, the other two sides are divided in the same ratio.
(ii) Area Theorem: The ratio of the areas of two similar triangles is equal to the square of the ratio of their corresponding sides.
(iii) Pythagoras Theorem: In a right triangle, the square of the hypotenuse is equal to the sum of the squares of the other two sides.
(iv) Converse of Pythagoras Theorem: In a triangle, if the square of one side is equal to the sum of squares of other two sides then the angle opposite to the first side is a right angle.
Important Questions for Practice
- In a triangle PQR, N is a point on PR such that Q N is perpendicular to PR. If PN.NR = QN², prove that angle PQR = 90°.
- Is the triangle with sides 12 cm, 16 cm and 18 cm a right triangle? Give reason.
- It is given that ∆DEF ~ ∆RPQ. Is it true to say that angle D = angle R and angle F = angle P?
- If the corresponding Medians of two similar triangles are in the ratio 5:7, then find the ratio of their sides.
- A right angled triangle has its area numerically equal to its perimeter. The length of each side is an even number and the hypotenuse is 10 cm. What is the perimeter of the triangle?
- An aeroplane leaves an airport and flies due west at a speed of 2100 km/hr. At the same time, another aeroplane leaves the same place at airport and flies due South at a speed of 2000 km/hr. How far apart will be the two planes after 1 hour?