NCERT Solutions for Class 10 Maths Chapter 6 Exercise 6.6 Triangles optional exercise free in English Medium and Hindi Medium form. Solutions for class 10 all subjects and sample papers with previous years papers are available in downloadable format. Download Exercise 6.1 or Exercise 6.2 or Exercise 6.3 or Exercise 6.4 or Exercise 6.5 in PDF form or view online.
NCERT Solutions for Class 10 Maths Chapter 6 Exercise 6.6
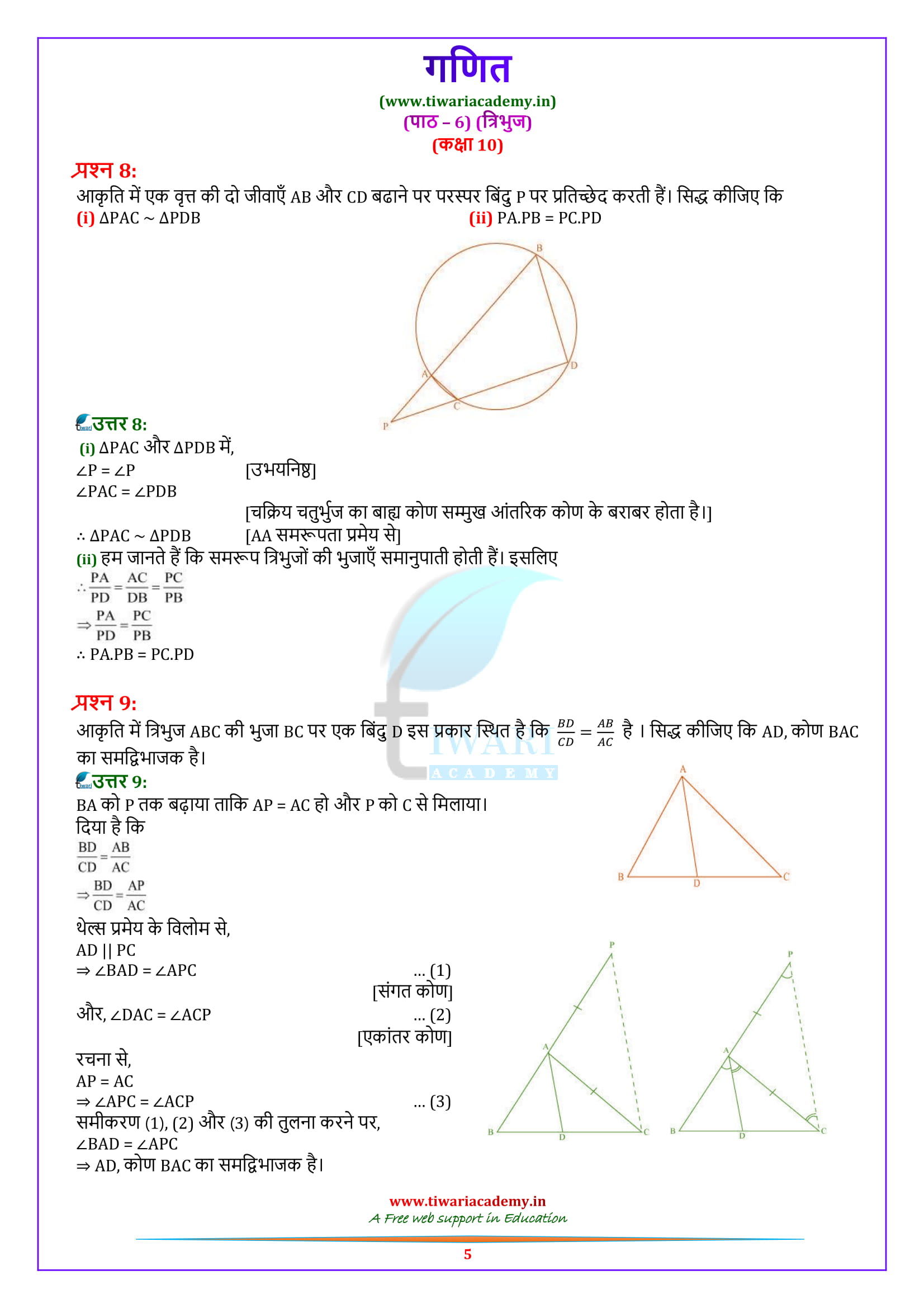
If you need solutions in Hindi, Click for Hindi Medium solutions of 10 Maths Exercise 6.6
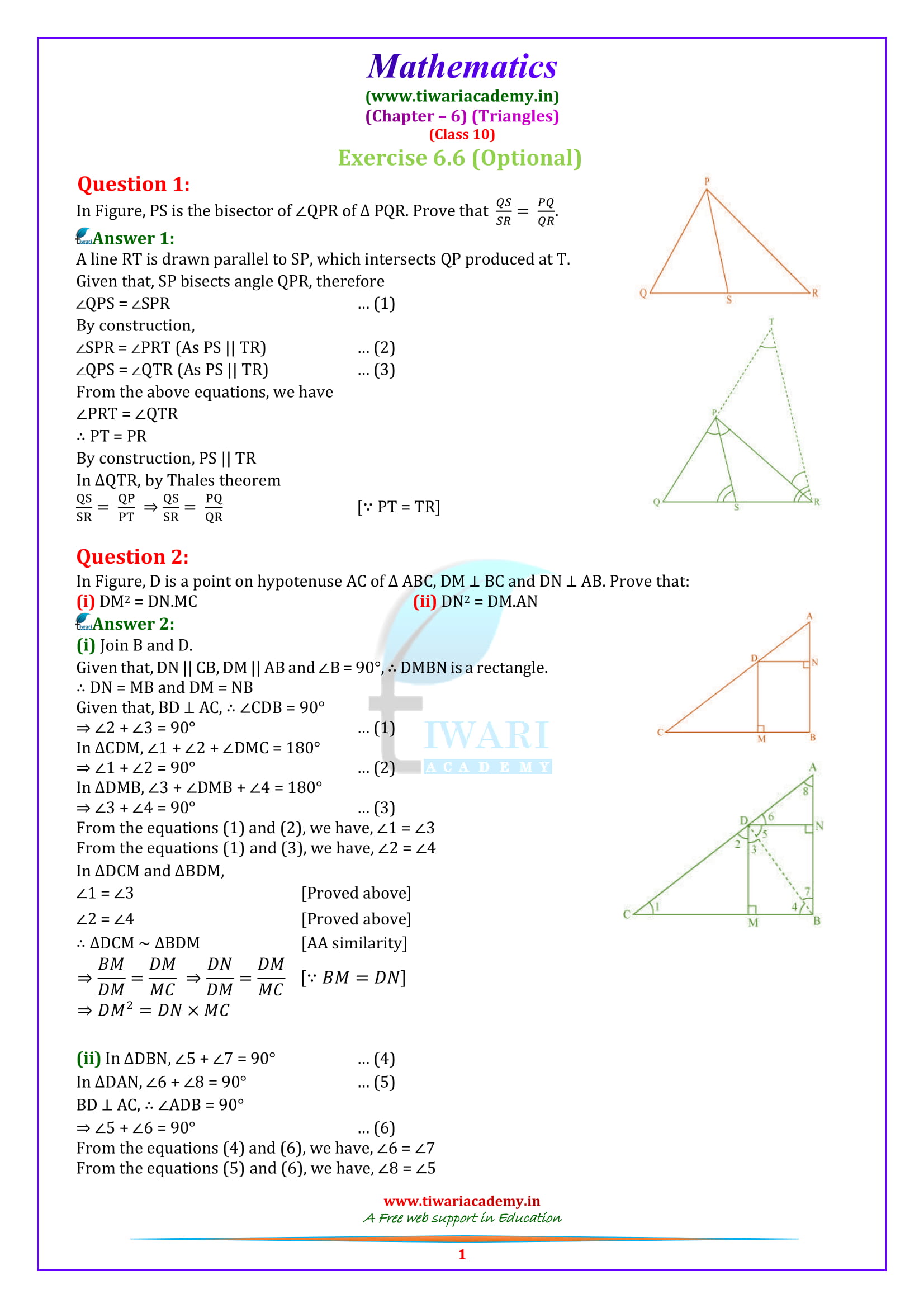
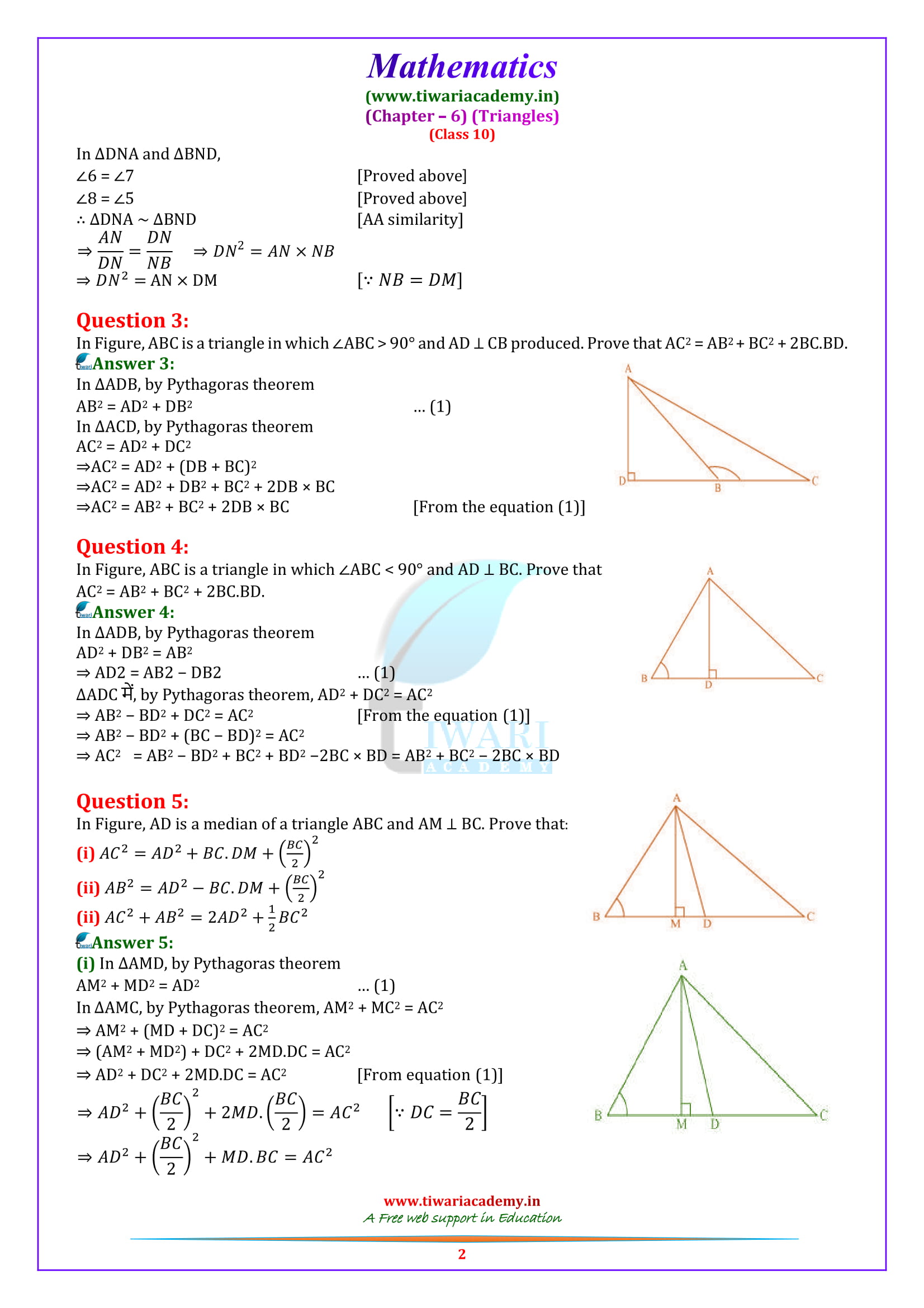
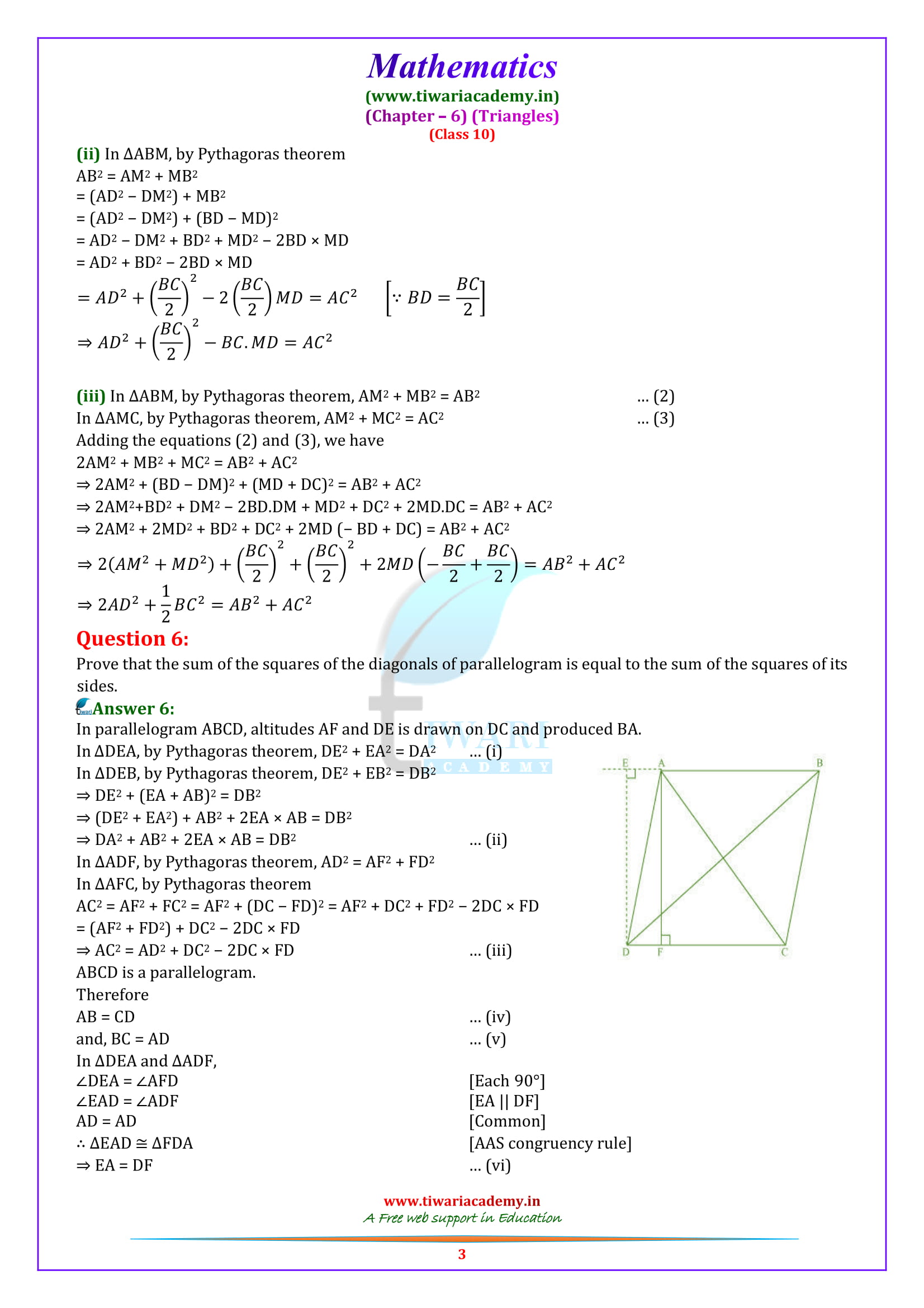
Class 10 Maths Exercise 6.6 Solutions in Hindi Medium



To get the solutions in English, Click for English Medium solutions.
Triangles – Optional Exercise (Containing HOTS Questions)
Important Questions for Practice
- Find the altitude of an equilateral triangle of side 8 cm.
- If ∆ABC ~ ∆DEF, AB = 4 cm, DE = 6 cm, EF = 9 cm and FD = 12 cm, find the perimeter of ∆ABC.
- Corresponding sides of two similar triangles are in the ratio of 2:3. If the area of the smaller triangle is 48 cm², find the area of the larger triangle.
- A 15 metres high tower casts a shadow 24 metres long at a certain time and at the same time, a telephone pole casts a shadow 16 metres long. Find the height of the telephone pole.
- It is given that ∆ABC ~ ∆EDF such that AB = 5 cm, AC = 7 cm, DF= 15 cm and DE = 12 cm. Find the lengths of the remaining sides of the triangles.
- Prove that if a line is drawn parallel to one side of a triangle to intersect the other two sides, then the two sides are divided in the same ratio.
- A 5 m long ladder is placed leaning towards a vertical wall such that it reaches the wall at a point 4 m high. If the foot of the ladder is moved 1.6 m towards the wall, then find the distance by which the top of the ladder would slide upwards on the wall.
- A street light bulb is fixed on a pole 6 m above the level of the street. If a woman of height 1.5 m casts a shadow of 3 m, find how far she is away from the base of the pole.
- O is the point of intersection of the diagonals AC and BD of a trapezium ABCD with AB is parallel DC. Through O, a line segment PQ is drawn parallel to AB meeting AD in P and BC in Q. Prove that PO = QO.
- Prove that the area of the equilateral triangle drawn on the hypotenuse of a right angled triangle is equal to the sum of the areas of the equilateral triangles drawn on the other two sides of the triangle.