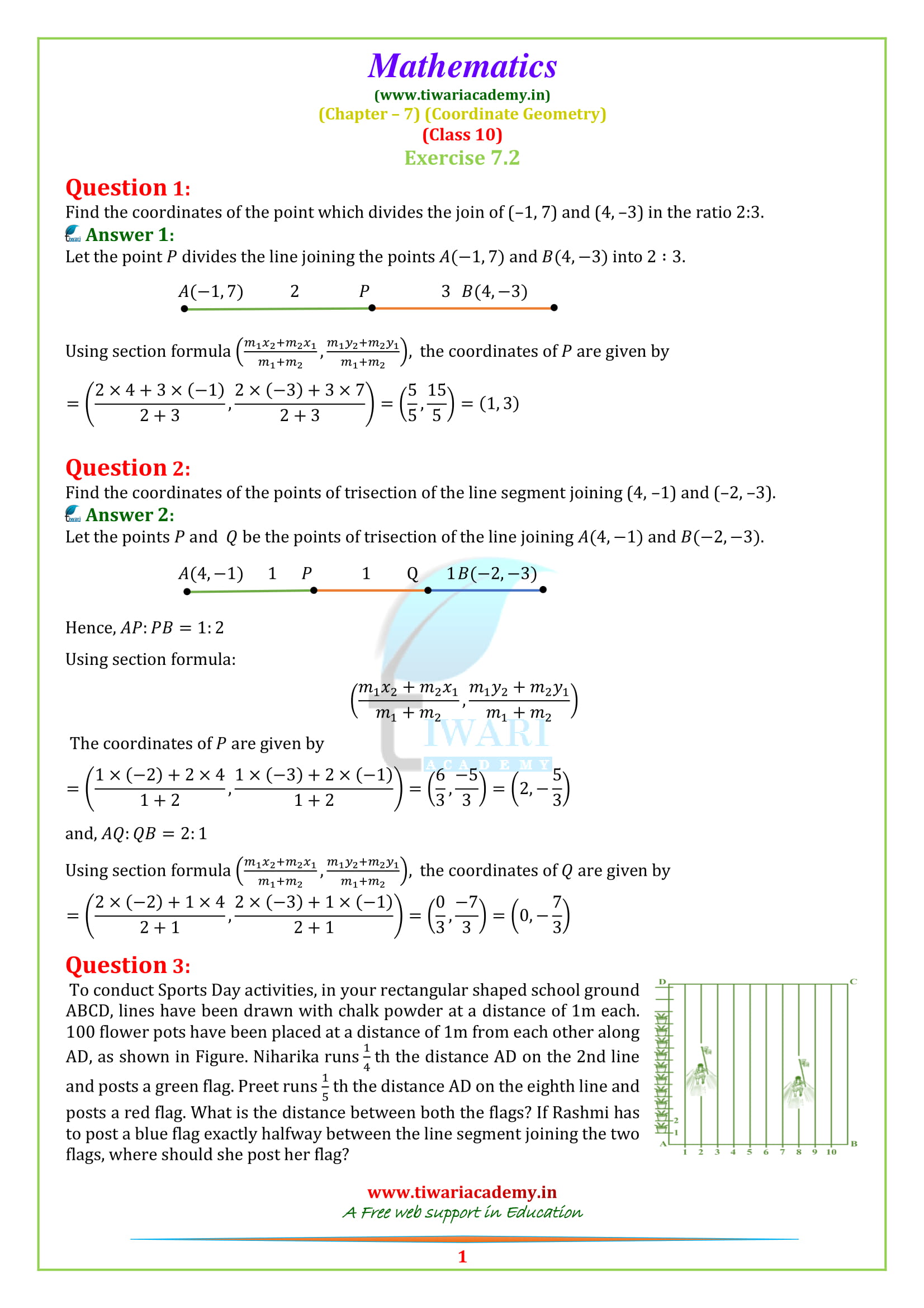
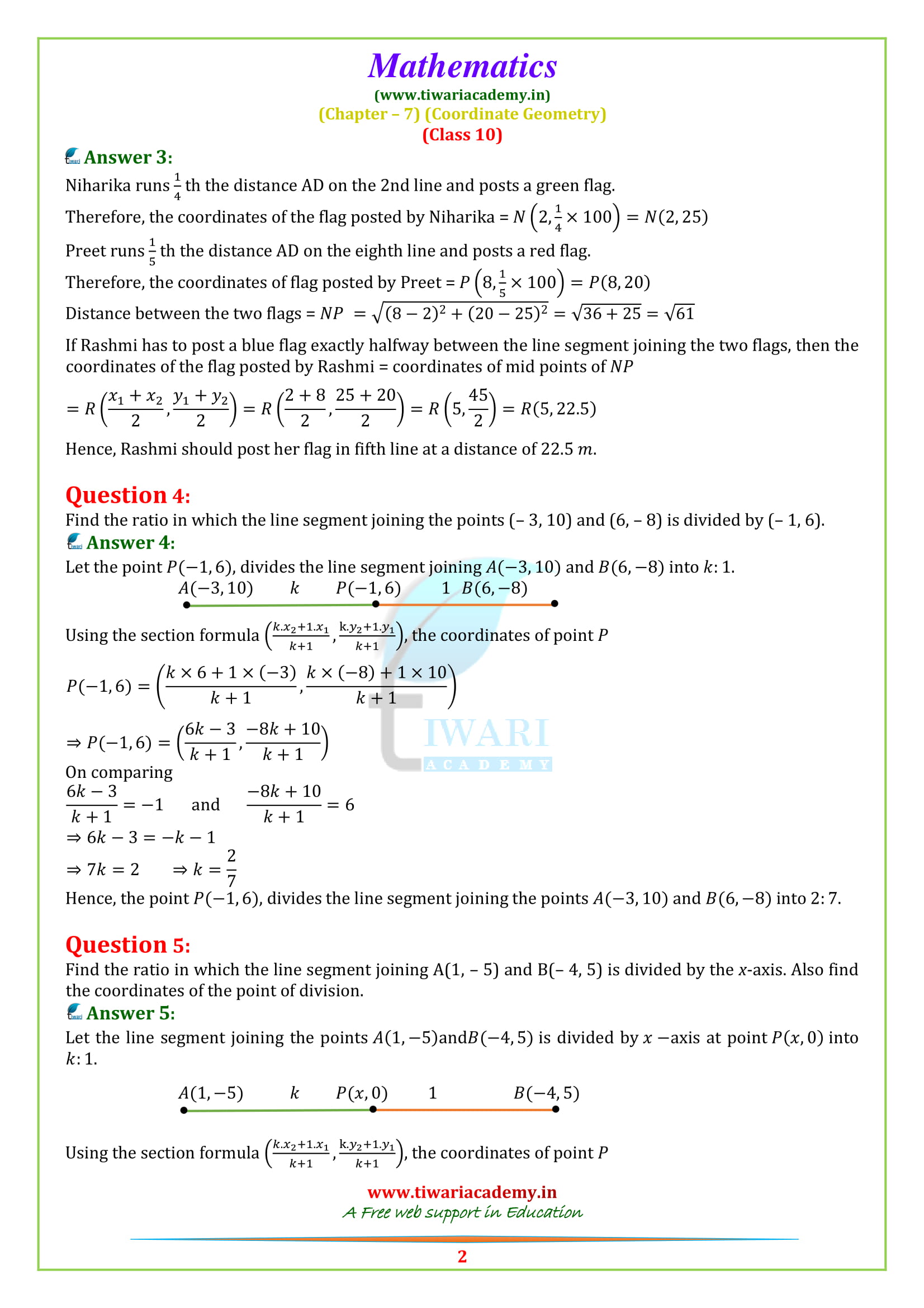
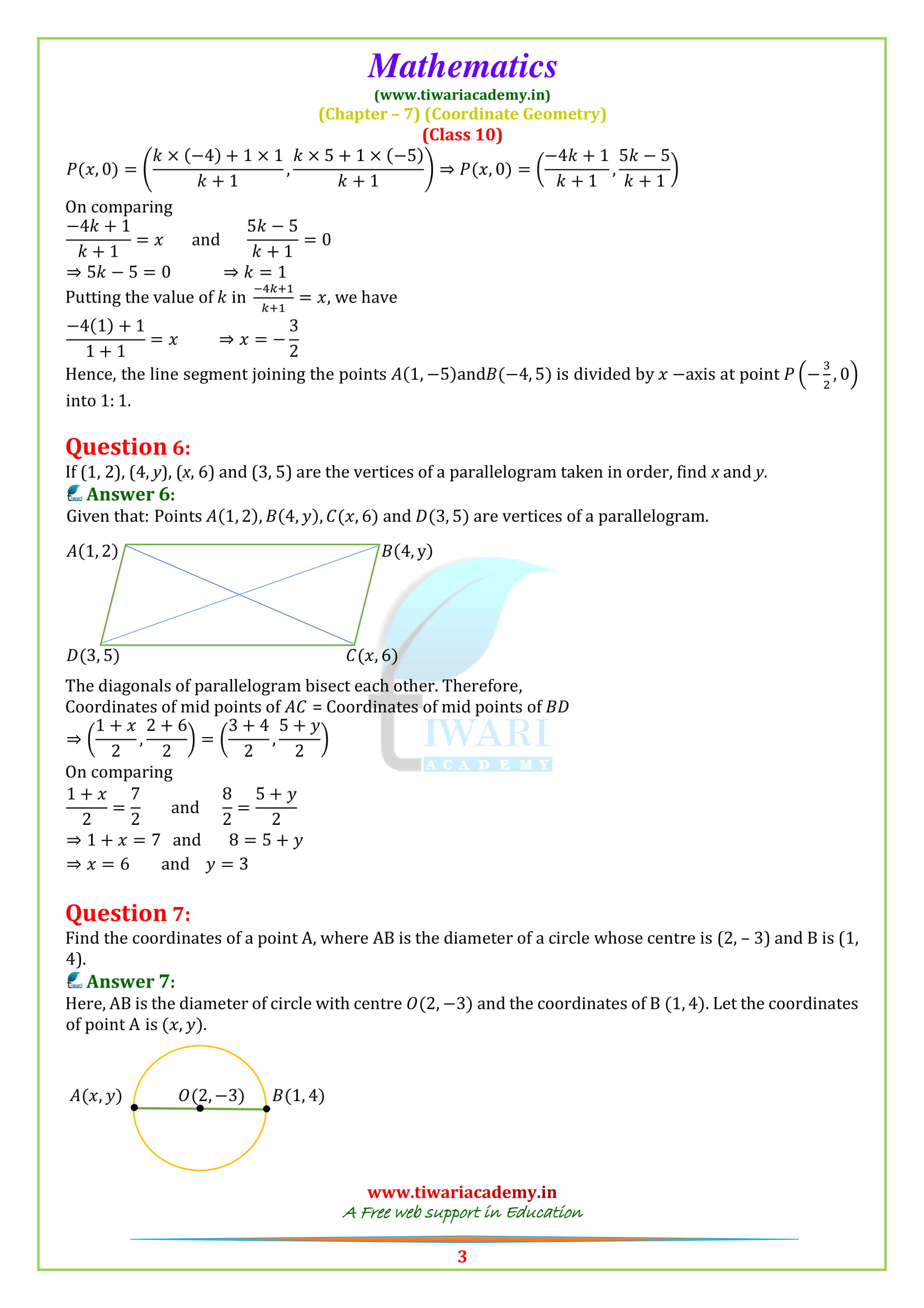
NCERT Solutions for Class 10 Maths Chapter 7 Exercise 7.2 Coordinate geometry in English Medium and Hindi Medium online study, download Exercise 7.1 or Exercise 7.3 or Exercise 7.4 also in in PDF form to use offline. Solutions of 10th maths other chapters are also given in PDF form. Click here to download NCERT books for class 10.
NCERT Solutions for Class 10 Maths Chapter 7 Exercise 7.2
If you need solutions in Hindi, Click for Hindi Medium solutions of 10 Maths Exercise 7.2

Class 10 Maths Exercise 7.2 Solutions in Hindi Medium



To get the solutions in English, Click for English Medium solutions.
Important questions with answers of Coordinate Geometry Exercise 7.2
- If P (a, 4) is the midpoint of the line segment joining the points Q (-6, 5) and R (-2, 3), then find the value of a. [Answer: -12]
- A line intersects y –axis and x-axis at the points P and Q respectively. If (2, -5) is the mid-point of PQ, then find the coordinates of P and Q respectively. [Answer: (0, – 10) and (4, 0)]
- Find the rational number which the y – axis divides the segment joining (-3, 6) and (12, -3). [Answer: 1/4]
- Find the coordinates of a point A, where AB is diameter of a circle whose Centre is (2, -3) and B is (1, 4). [Answer: (3, – 7)]
- If A (-2, 4), B (0, 0), C (4, 2) are the vertices of a ΔABC, then find the length of median through the vertex A. [Answer: 5 units]
- Point P divides the line segment joining the points A (2, 1) and B (5, -8) such that AP: AB = 1:3. If P lies on the line 2x – y + k = 0, then find the value of k. [Answer: -8]
- Find the ratio in which the line 2x + 3y = 10 divides the line segment joining the points (1, 2) and (2, 3). [Answer: 2:3]
- Find the area of the triangle formed by joining the midpoints of the sides of the triangle whose vertices are (0,-1), (2, 1) and (0, 3). Find the ratio of this area to the area of the given triangle. [Answer: 1:4]
- Determine the ratio in which the point P (a, -2) divides the line joining of points (-4, 3) and B (2, – 4). Also find the value of a. [Answer: a = 2/7]
- If the point C (-1, 2) divides internally the line segment joining A (2, 5) and in the ratio 3:4. Find the Co-ordinates of B. [Answer: B (-5, -2)]
- Find the ratio in which the Y-axis divides the line segment joining the points (5, -6) and (-1,-4). Also find the coordinates of the point of division. [Answer: 5:1, (0, -13/3]
- Find the ratio in which the point P (x, 2) divides the line- segments joining the points A (12, 5) and B (4, -3). Also, find the value of x. [Answer: 3:5, x = 9]
- In what ratio does the point (-4, 6) divides the line segment joining the points A (-6, 10) & B (3, -8). [Answer: 2/7]