Free to use NCERT Solutions for Class 10 Maths Chapter 8 Exercise 8.2 introduction to trigonometry (trigo) in English Medium and Hindi Medium downloadable form or online. NCERT Books Solutions for 10 all subjects with practice papers based on latest CBSE Sample Papers. Download Exercise 8.1 or Exercise 8.3 or Exercise 8.4 also in PDF form if you don’t want to use it online.
NCERT Solutions for Class 10 Maths Chapter 8 Exercise 8.2
If you need solutions in Hindi, Click for Hindi Medium solutions of 10 Maths Exercise 8.2
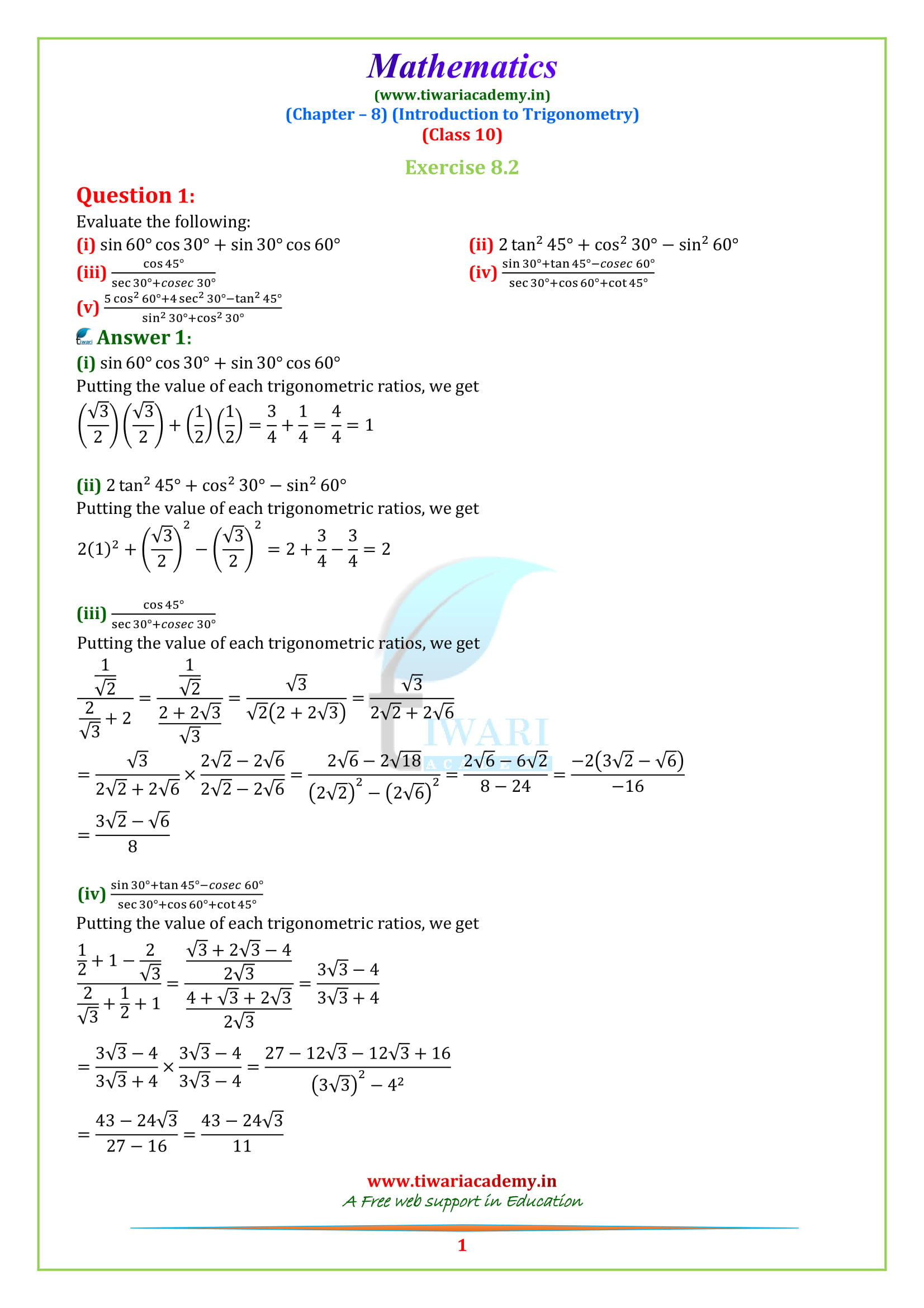
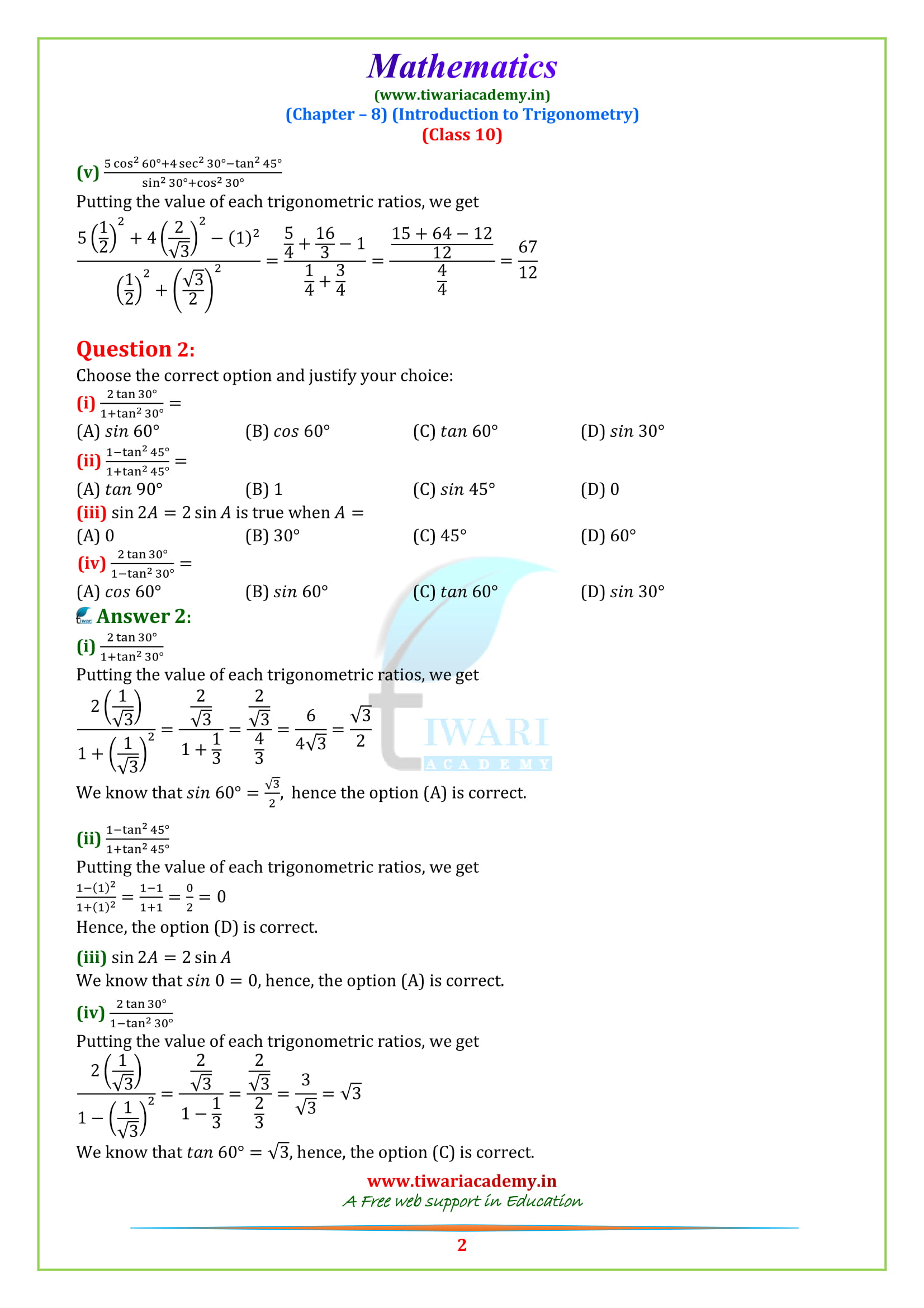

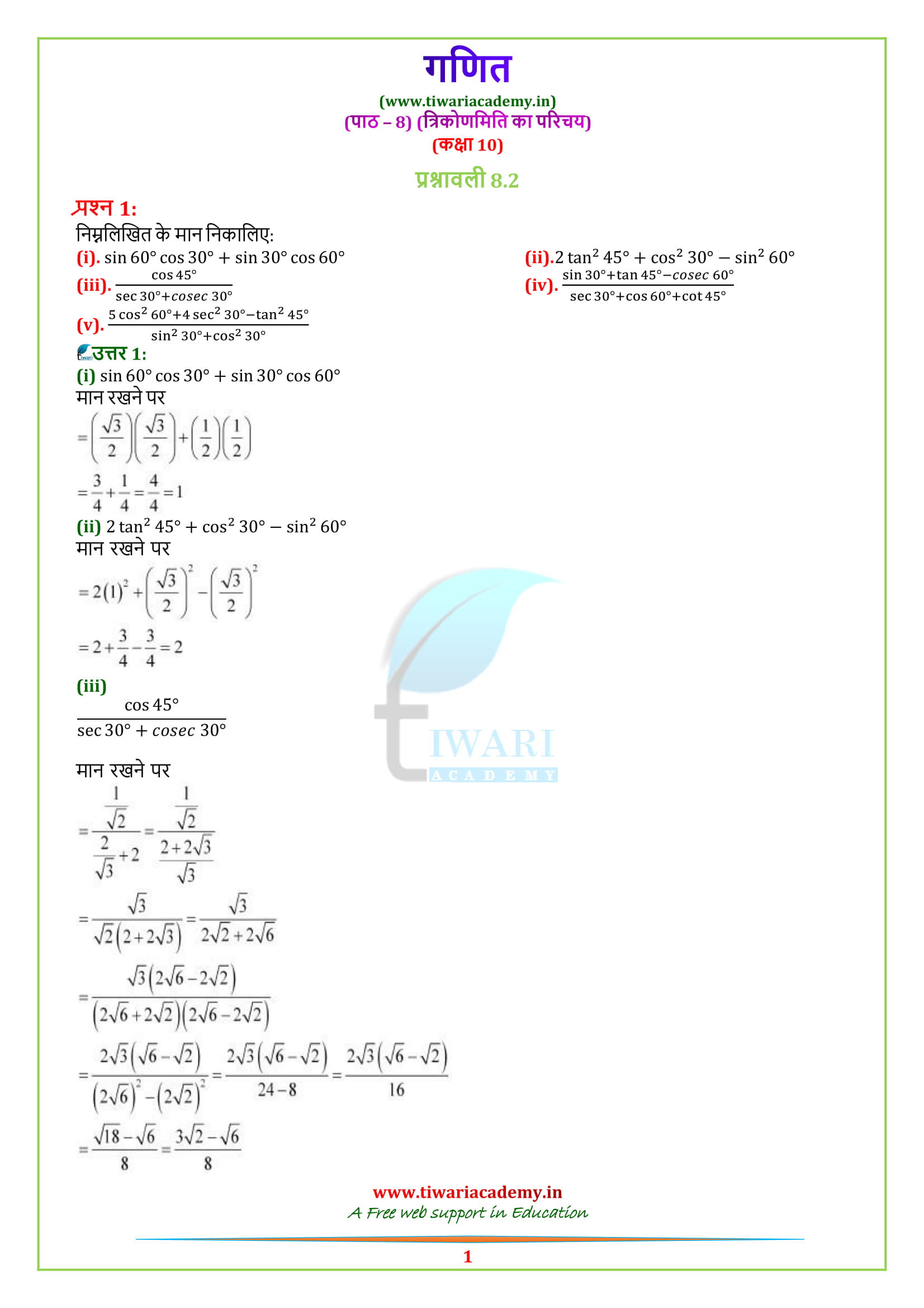
Class 10 Maths Exercise 8.2 Solutions in Hindi Medium

To get the solutions in English, Click for English Medium solutions.
Introduction to Trigonometry
Important Questions with answers for Practice
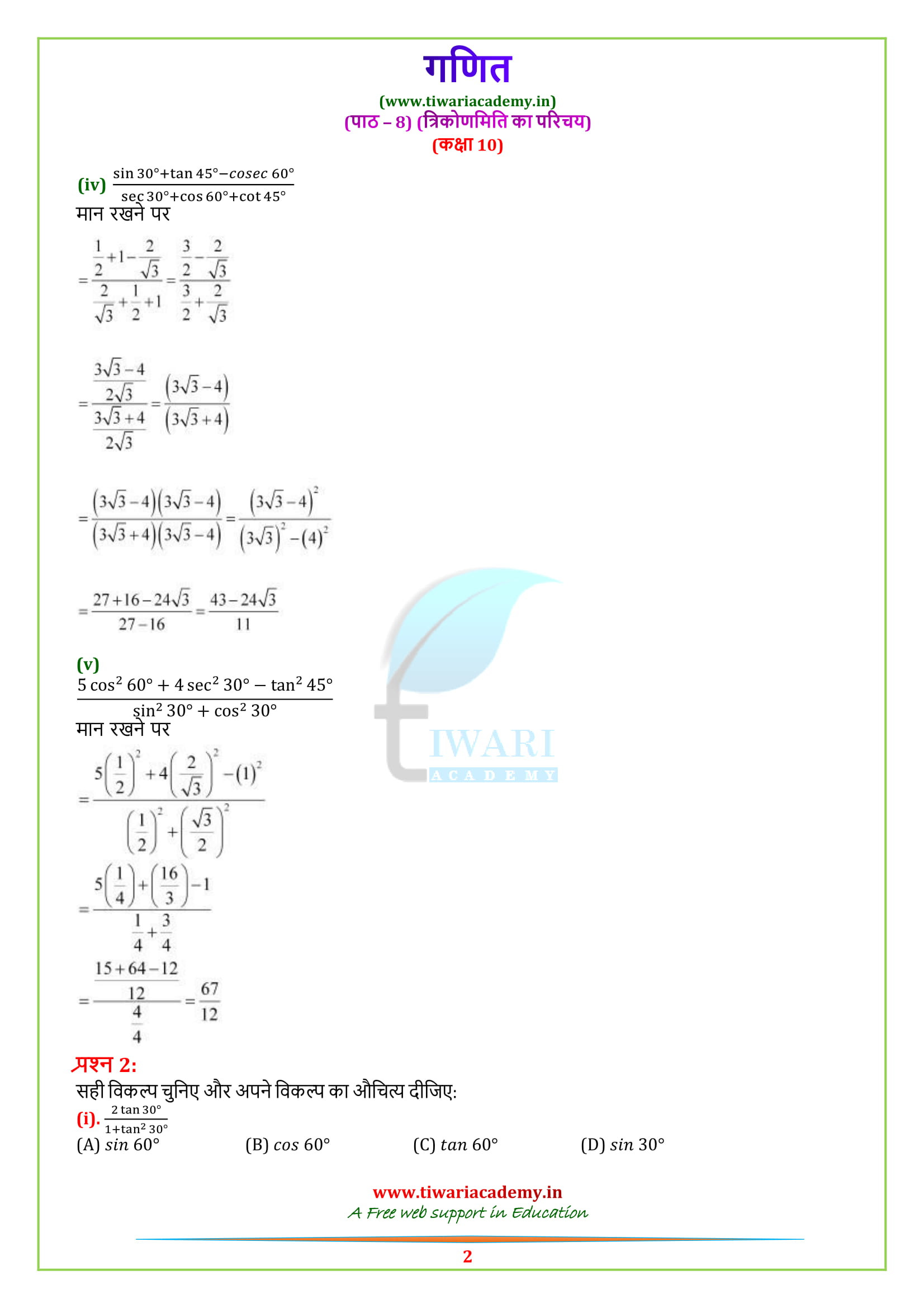
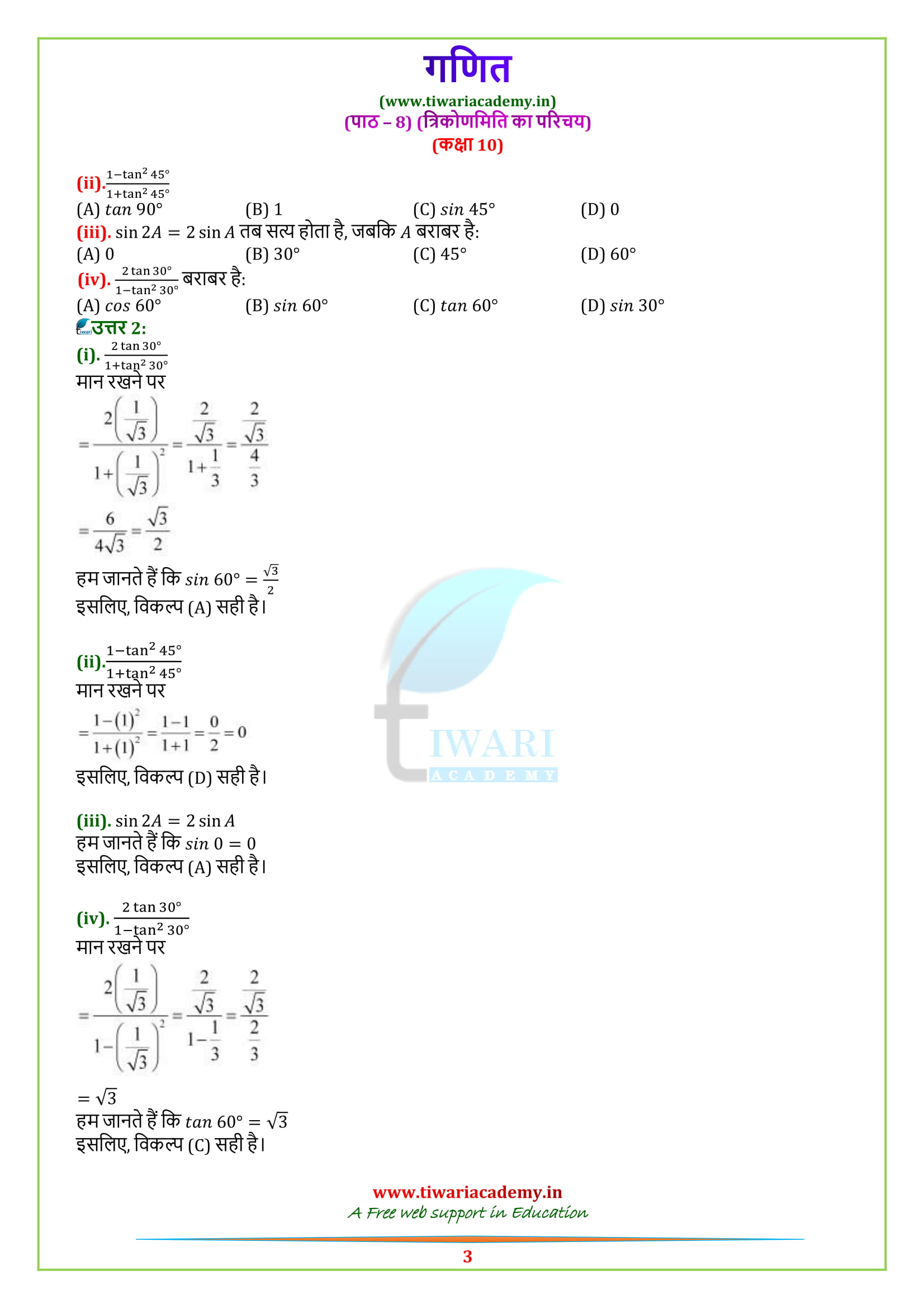
Question 1: In ΔABC, angle B = 90⁰, BC = 5 cm, AC – AB = 1, Evaluate [1+sin C]/[1+cos C]. [Answer: 25/18]
Question 2: If cosec A = 5/4, verify that [tan A] / [1+tan2 A] = [sin A] / [sec A].
Question 3: If 15 cotA = 8, find all other trigonometric ratio of angle A and verify the result cos2A = 1 – sin²A.
Question 4: Find the value of cos A cot A cosec A, if sin A = 1/4. [Answer: 15]
Question 5: If sin (A + B) = cos (A – B) = [√3]/2 and A, B (A > B) are acute angles, find the values of A and B. [Answer: A = 45⁰ and B = 15⁰]
Question 6: Evaluate: 2 cosec²30⁰ + 3 sin²60⁰ – 3/4 tan²30⁰. [Answer: 10]
Question 7: If sin x + cos y = 1; x = 30⁰ and y is acute angle, find the value of y. [Answer: 60⁰]
Question 8: Evaluate: [tan²45⁰] / [sin2 45⁰ + cos2 45⁰]. [Answer: 1]
Question 9: If cos α = 1/2 and tan β = 1/√3, find sin (α + β), where α and β are both acute angles. [Answer: 1]
Question 10: Find the value of θ, if tan 9θ = sin 45⁰ cos 45⁰ + sin 30⁰, given that θ is acute. [Answer: 5⁰]
Question 11: If 2(cos2 45⁰ + tan2 60⁰) –x(sin2 45⁰ – tan2 30⁰) = 6, find the value x. [Answer: x = -6]
Question 12: Given that sin (A + B) = sin A cos B + cos A sin B, find the value of sin 75⁰. [Answer: [√3 + 1] / 2√2]
Question 13: Derive the value of sin 30⁰ and cos 60⁰, geometrically. [Answer: sin 30⁰ = 1/2 and cos 60⁰ = 1/2]
Question 14: If 2 sin (3x – 15)⁰ = √3, find the value of sin2 (2x + 10)⁰ + tan2 (x + 5)⁰. [Answer: 13/12]
Question 15: If A = 30⁰. Verify that sin 2A = [2tan A] / [1 + tan2 A].
Question 16: In an acute-angle triangle ABC, if sin (A + B – C) = 1/2 and cos (B + C – A) = 1/√2, find angle A, B and C. [Answer: Angle A = 67 1/2⁰, angle B = 37 1/2⁰, angle C = 75⁰]