NCERT Solutions for Class 10 Maths Chapter 9 Exercise 9.1 Question number 9, 10, 11, 12, 13, 14, 15 and 16 in English Medium and Hindi Medium PDF complete description. Download Exercise 9.1 Questions 1 to 8 in PDF or view online without downloading. NCERT solutions for class 10 are available in PDF form.
NCERT Solutions for Class 10 Maths Chapter 9 Exercise 9.1
If you need solutions in Hindi, Click for Hindi Medium solutions of 10 Maths Exercise 9.1
Class 10 Maths Exercise 9.1 Solutions in Hindi Medium
To get the solutions in English, Click for English Medium solutions.
Important questions based on Height and Distance
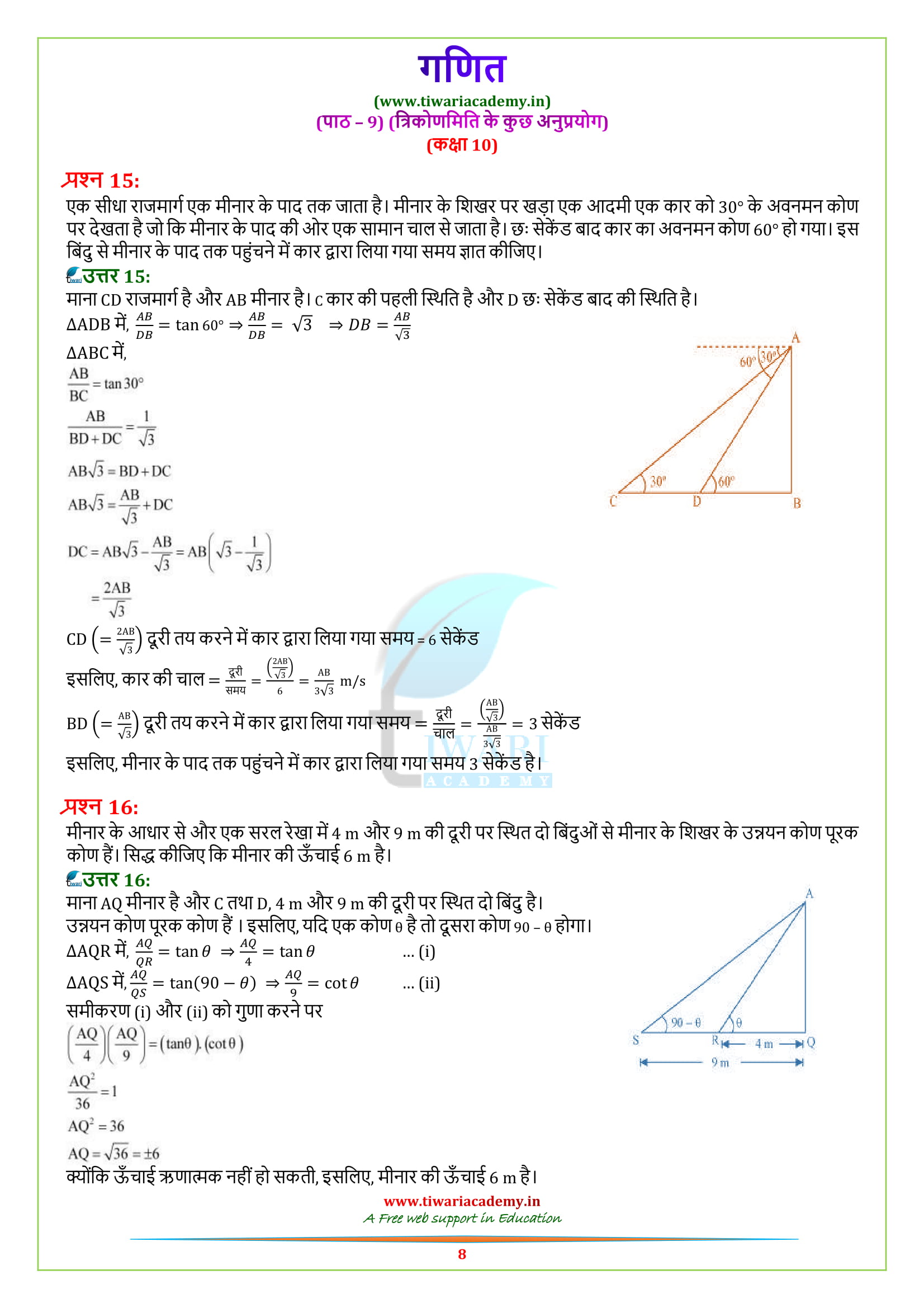
- The angle of elevation of an aeroplane from a point on the ground is 45o. After flight for 15 seconds the elevation changes to 30. If the aeroplane is flying at a height of 3000m. Find the speed of the aeroplane. [Answer: 527.4km/h]
- The angle of elevation of a cloud from a point h metres above a lake is α and the angle of derpression of its reflection in the lake is β. Prove that the height of the cloud is (h(tan〖β+tanα)〗)/(tanβ-tanα) metres.
- From the top of a light house, the angles of depression of two ships on opposite sides of it are observed to be α and β. If the height of the light house is h metres and the line joining the ships passes through the foot of the lighthouse, show that the distance between ships is (h(tan〖α+tanβ)〗)/(tanα.tanβ) metres.
- The angle of elevation of the top of towers from points p and q at distances of a and b respectively from the base and in the same straight line with it are complementary. Prove that the height of the tower is √ab.
- A man standing on the deck of a ship which is 10m above the water level observes the angle of elevation of the top of a hill as 60o and the angle of depression of the base of the hill is 30o. Calculate the distance of the hill from the ship and the height of the hill.
- The angle of elevation of the top of the tower as observed from a point on the ground is α and on moving ‘a’ m towards the tower, the angle of elevation is β. Prove that the height of the tower is (a tan〖α .tanβ 〗)/tan〖β-tanα 〗 .
- A person standing on the bank of a river observes that the angle of elevation of the top of a tree standing on the opposite bank is 60o. When he moves 40m away from the bank, he finds that angle of elevation to be 30o. Find the height of the tree and the width of the river. [use √3=1.732]