Download NCERT Solutions for Class 9 Maths Chapter 12 Exercise 12.1 and Exercise 12.2 of Heron’s Formula in English Medium and Hindi Medium free to use online. NCERT Solutions for Class 9 all subjects and other chapters of maths are also given in PDF format. NCERT Books in ZIP format and NCERT Books in PDF format are available to download.
NCERT Solutions for Class 9 Maths Chapter 12
If you need solutions in Hindi, Click for Hindi Medium solutions of 9 Maths Chapter 12


NCERT Solutions 9 Maths: Main Page
9 Maths Chapter 12 Exercise 12.1 & Exercise 12.2 in Hindi



NCERT Solutions 9 Maths: Main Page
To get the solutions in English, Click for English Medium solutions.
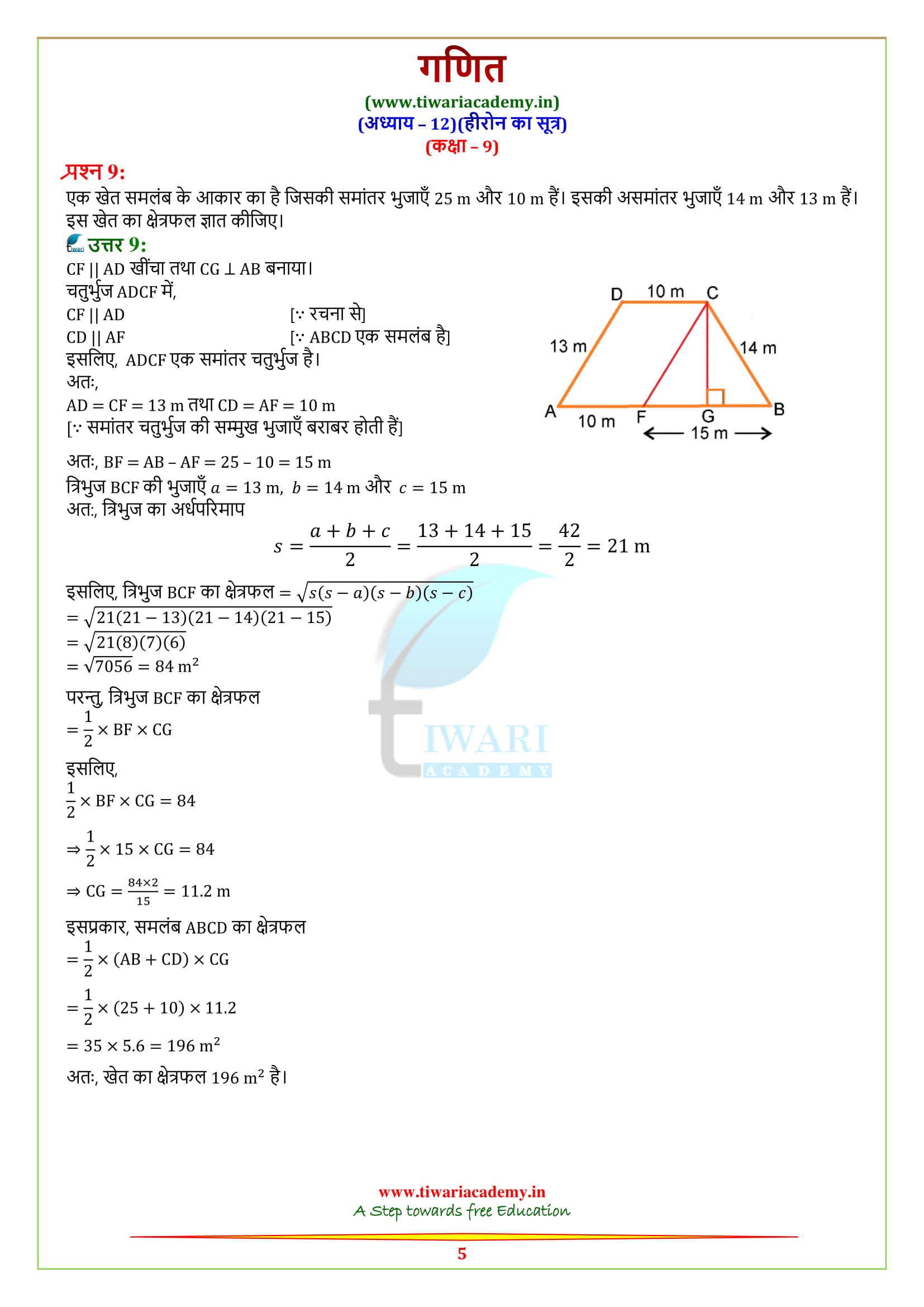
Class 9 Maths – Heron’s Formula
Important Extra Questions with Answers
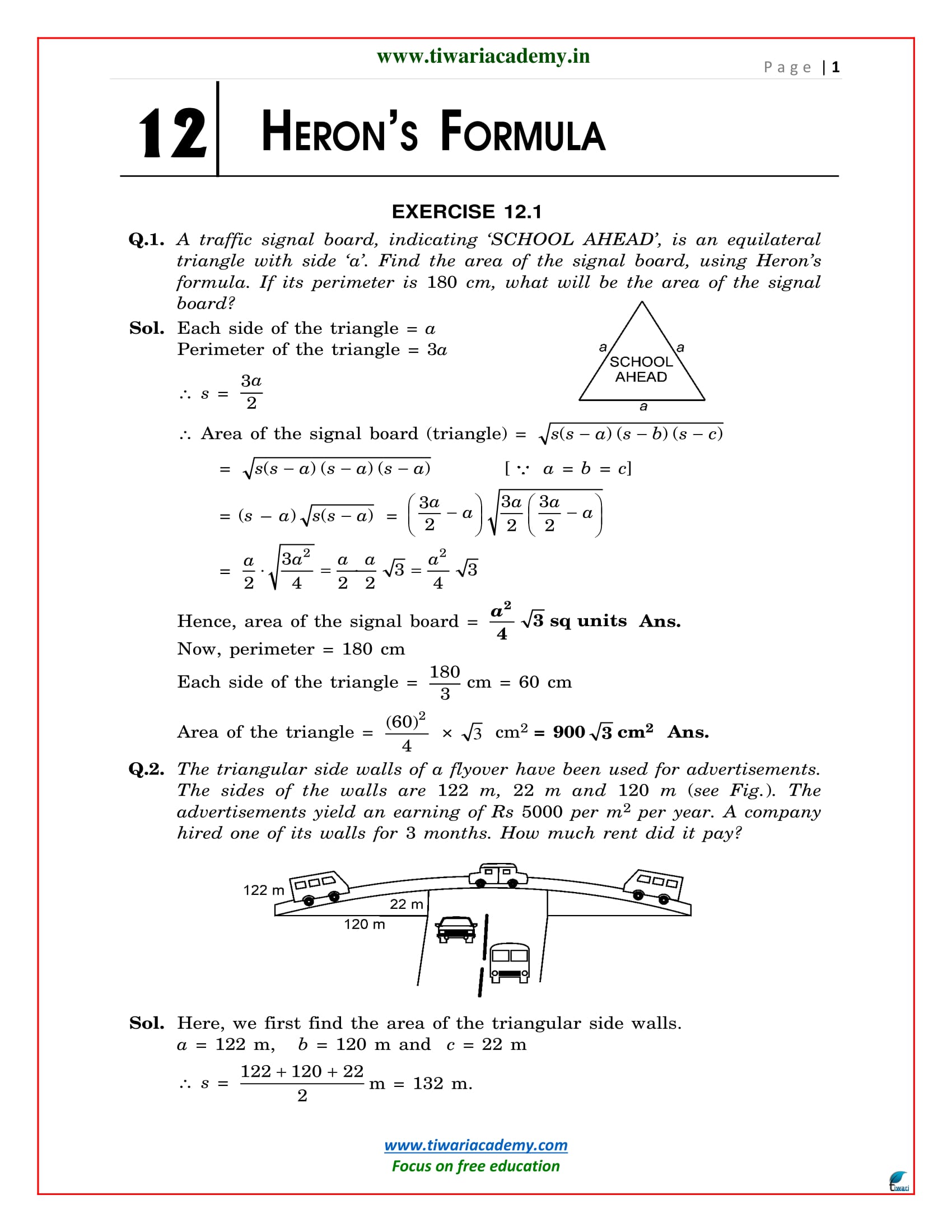
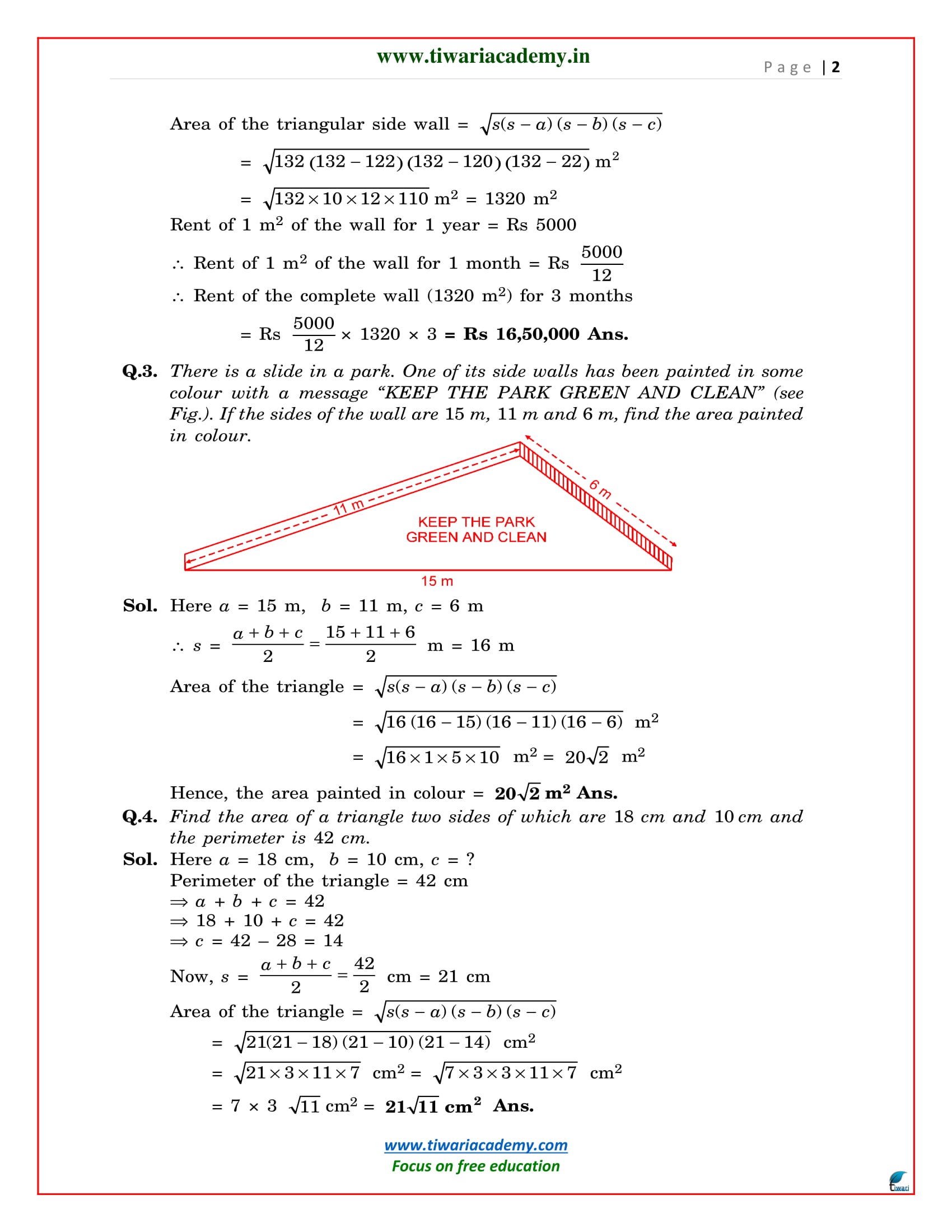
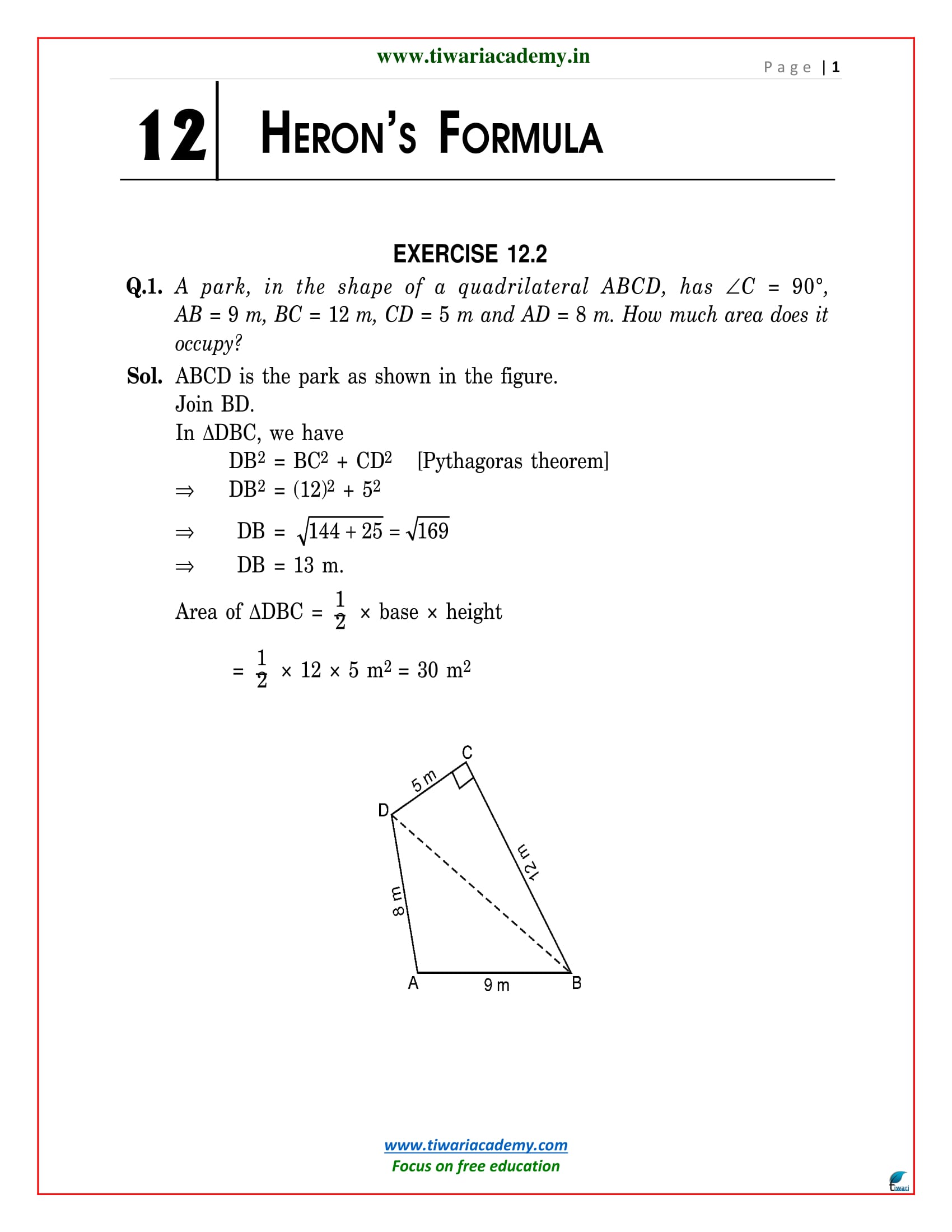
- Find the area of a triangle whose base and altitudes are 8 cm and 5 cm. [Answer: 20 cm²]
- Find the area of an equilateral triangle whose sides are 4 cm each. [Answer: 4√3 cm²]
- If sum of two sides of a triangle is 17 cm and its perimeter is 30 cm, then what is the length of third side? [Answer: 13 cm]
- If perimeter of a triangle is 24 cm and sides are in the ratio 2:1:3, then find the longest side? [Answer: 12 cm]
- If each sides of a triangle is doubled then how many times the area of triangle increased? [Answer: 3 times]
- If area of a triangle is 50 cm² and one of its sides is 10 cm then find the length of corresponding altitude. [Answer: 10 cm]
- The area of an equilateral triangle is 16√3 cm² then what will be the length of each side of that triangle? [Answer: 8 cm]
- A square has each side of 5 cm. Find the length of one of its diagonals. [Answer: 5√2 cm]
- If a parallelogram has length is 10 cm and 8 cm then find the area of a triangle made by its diagonal. [Answer: 40 cm²]
- If area of a triangle is doubled to its area then what is the percentage increased in the area of triangle? [Answer: 100%]
- If one side of a triangle is 9.5 m and its corresponding altitude is 12 m then what will be the area of triangle. [Answer: 57 m²]
- The ratio between the sides of a triangle are 3:5:7 and its perimeter is 300 cm find the sides of triangle. [Answer: 60 cm, 100 cm, 140 cm]
- Find the cost of fencing the ground in the form of a triangle with sides 16 m, 12 m and 18 m. The rate of fencing is ₹ 25 per meter. [Answer: ₹ 1150]
- Find the area of isosceles triangle whose non equal sides of 12 cm having the corresponding altitude is 7.5 cm. [Answer: 45 cm²]
- In a right angled triangle the sides make the right angle are 10 cm and 24 cm. Find the area of triangle. [Answer: 120 cm²]
- If in a triangle AB = 15 cm, BC = 14 cm and AC = 13 cm. Find the area of ∆ABC and hence its altitude on BC. [Answer: 84 cm², 12 cm]
- The diagonals of a rhombus are 10 cm and 24 cm. Find its area and perimeter. [Answer: 120 cm², 52 cm]
- Find the area of a triangle or region whose sides are 1.6 m, 1.2 m and 2.0 m. [Answer: 0.96 m²]
- The perimeter of a triangle shaped ground is 420 m and its sides are in the ratio 6:7:8. Find the area of ground. [Answer: 2100√15 m²]
- Find the area of rhombus whose perimeter is 100 m and one of whose diagonal is 30 m. [Answer: 300 m²]
- The sides of a triangle 5 cm, 12 cm and 13 cm. Find the cost of painting on the triangle at the rate of ₹ 30 per cm². [Answer: ₹ 900]
- If perimeter of a triangle is x cm and its sides are p, q and r cm. What will be the area of triangle? Use the Heron’s formula.
- A Triangular Park ABC has sides 120 m, 80m and 50 m. A gardener Dhani Ram has to put a fence all around it and also plant some trees inside the garden to get clean air.
(i) Find the cost of fencing it at the rate of ₹ 50 per meter. Leaving space 5 cm wide for the gate on one side.
(ii) Find its area where Dhani Ram may plant the tree.
(iii) What values of Dhani Ram do you assess here? [Answer: (i) ₹ 12250, (ii) 375√15 m², (iii) Caring about environment clean air]
- A triangular hoarding of dimension 11m, 6 m and 15 m is used for commercial activities. The hoarding yield an earning of ₹ 5000 per m² per month. Calculate the total earning by the hoarding in a month. [Use √2 = 1.7] [Answer: ₹ 510000]